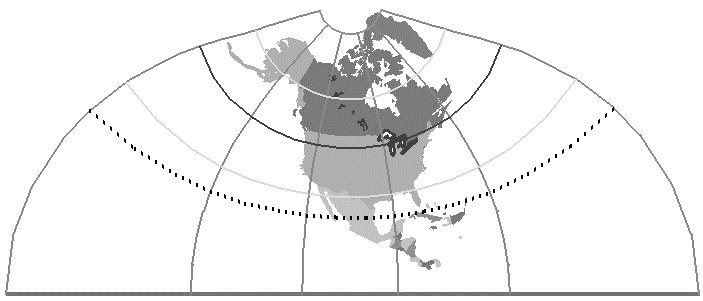
Transverse Mercator (TM)
A conformal projection, first presented by Lambert in 1772. Sometimes known as Gauss-Kruger or simply Gauss (when scale reduction factor is 1.0). In its ellipsoidal form is one of the most widely used projections in the world. It is used for official topographic mapping in many countries. In the United States, it is used with the State Plane Coordinate System for States with a north-south extent.
With the Transverse Mercator projection, most meridians and parallels are complex curves. The central meridian and meridians ninety degrees from the central meridian, as well as the Equator are straight lines.
The well known Universal Transverse Mercator (UTM) is a specific use of the Transverse Mercator with the specification of central meridians and a scale reduction factor of 0.9996 (a 1:2500 reduction).
MicroStation PowerDraft supports many variations of the transverse Mercator:
- Gauss-Kruger — Identical to Transverse Mercator when scale reduction is 1.0. Scale reduction factor does not appear as a parameter for this projection. This coordinate system exists for users who are used to the Gauss-Kruger projection terminology.
- Transverse Mercator — The plain Transverse Mercator applicable to a range of longitude approximately 6 degrees wide.
- Transverse Mercator Snyder Formulation — A similar Transverse Mercator that uses slightly different equations. The results are quite similar and virtually identical to the Transverse Mercator within any feasible limits of ground measurements. It is provided only as a means of obtaining consistent results with previously generated data using this method.
- Transverse Mercator Using BF Calculation — See description in Universal Transverse Mercator (UTM).
- Transverse Mercator Wisconsin County — See description of Lambert Wisconsin County in Lambert Conformal Conic.
- Transverse Mercator Minnesota DOT — See description of Lambert Minnesota County in Lambert Conformal Conic. This method can be mimicked by simply computing the appropriate local scale reduction factor with a plain Transverse Mercator.
- Transverse Mercator with Affine Post-Processor — Similar to Transverse Mercator projection but also allows an affine transform to take place after the projection has taken place.
- South-Oriented Transverse Mercator — A variation of Transverse Mercator mainly used in South Africa. It is similar to Transverse Mercator with the axis inverted but is provided as a separate projection method for ease of use.